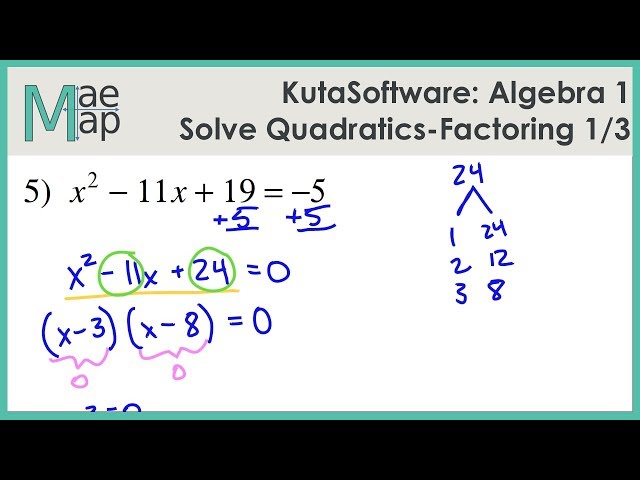
A quadratic equation is an equation of the second degree, meaning it contains a variable that is squared. Solving quadratic equations by factoring is a process of finding the values of the variable that make the equation true. To factor a quadratic equation, you need to find two numbers that add up to the coefficient of the x-term and multiply to the constant term. Once you have found these two numbers, you can factor the equation as (x + a)(x + b), where a and b are the two numbers you found.
Solving quadratic equations by factoring is an important skill because it can be used to solve a wide variety of problems. For example, quadratic equations can be used to find the roots of a polynomial, to solve problems involving the area of a rectangle, and to solve problems involving the Pythagorean theorem. Solving quadratic equations by factoring is also a relatively simple process, so it is a good place to start when learning how to solve quadratic equations.
There are a number of different ways to solve quadratic equations by factoring. One common method is to use the zero product property. The zero product property states that if the product of two numbers is zero, then at least one of the numbers must be zero. To use the zero product property to solve a quadratic equation, you need to factor the equation and then set each factor equal to zero. Solving each equation will give you the values of the variable that make the original equation true.
Solving Quadratic Equations by Factoring Worksheet
Solving quadratic equations by factoring is an important skill in algebra. This worksheet provides practice problems to help you master this skill.
- Factoring: Factoring is the process of finding two numbers that multiply to give the constant term and add to give the coefficient of the x-term.
- Zero Product Property: The zero product property states that if the product of two numbers is zero, then at least one of the numbers must be zero.
- Solving: Once you have factored the quadratic equation, you can solve it by setting each factor equal to zero and solving for x.
To practice solving quadratic equations by factoring, complete the problems on the worksheet. You can check your answers using the answer key provided.
Factoring
Factoring is an essential step in solving quadratic equations by factoring. Without factoring, it would be much more difficult to find the roots of a quadratic equation. Factoring allows us to break down the quadratic equation into two simpler equations that are easier to solve.
For example, consider the quadratic equation x^2 + 5x + 6 = 0. We can factor this equation as (x + 2)(x + 3) = 0. This means that either x + 2 = 0 or x + 3 = 0. Solving these two equations gives us the two roots of the original quadratic equation, which are x = -2 and x = -3.
The process of factoring can be used to solve any quadratic equation. It is a valuable skill that can be used to solve a variety of problems in algebra and beyond.
Zero Product Property
The zero product property is a fundamental property of real numbers that has many applications in mathematics, including solving quadratic equations by factoring.
- Using the zero product property to solve quadratic equations: The zero product property can be used to solve quadratic equations by factoring. To do this, we first factor the quadratic equation so that it is in the form (ax + b)(cx + d) = 0. Then, we use the zero product property to conclude that either ax + b = 0 or cx + d = 0. Solving each of these equations gives us the two solutions to the original quadratic equation.
- Example: Solve the quadratic equation x^2 + 5x + 6 = 0. Factoring the left-hand side of the equation, we get (x + 2)(x + 3) = 0. Using the zero product property, we conclude that either x + 2 = 0 or x + 3 = 0. Solving each of these equations gives us the two solutions to the original quadratic equation, which are x = -2 and x = -3.
The zero product property is a powerful tool that can be used to solve a variety of problems in algebra, including quadratic equations. It is a fundamental property of real numbers that has many applications in mathematics.
Solving
Solving quadratic equations by factoring is a valuable skill that can be used to solve a variety of problems. It is a relatively simple process, but it is important to understand the steps involved in order to solve quadratic equations correctly.
- Factoring the quadratic equation: The first step in solving a quadratic equation by factoring is to factor the equation. This involves finding two numbers that multiply to give the constant term and add to give the coefficient of the x-term. Once you have factored the equation, you can set each factor equal to zero and solve for x.
- Setting each factor equal to zero: Once you have factored the quadratic equation, you can set each factor equal to zero. This will give you two equations, which you can solve for x.
- Solving for x: Solving each equation for x will give you the two solutions to the original quadratic equation. These solutions can be either real numbers or complex numbers.
Solving quadratic equations by factoring is a powerful tool that can be used to solve a variety of problems. It is a relatively simple process, but it is important to understand the steps involved in order to solve quadratic equations correctly.
Solving Quadratic Equations by Factoring Worksheet FAQs
This FAQ section provides answers to some of the most common questions about solving quadratic equations by factoring. If you have any other questions, please feel free to leave a comment below.
Question 1: What is factoring?
Answer: Factoring is a process of finding two numbers that multiply to give the constant term and add to give the coefficient of the x-term in a quadratic equation.
Question 2: How do I solve a quadratic equation by factoring?
Answer: To solve a quadratic equation by factoring, you first need to factor the equation. Once you have factored the equation, you can set each factor equal to zero and solve for x.
Question 3: What is the zero product property?
Answer: The zero product property states that if the product of two numbers is zero, then at least one of the numbers must be zero.
Question 4: How do I use the zero product property to solve a quadratic equation?
Answer: To use the zero product property to solve a quadratic equation, you first need to factor the equation. Once you have factored the equation, you can set each factor equal to zero and solve for x.
Question 5: What are the benefits of solving quadratic equations by factoring?
Answer: Solving quadratic equations by factoring is a valuable skill that can be used to solve a variety of problems. Factoring can help you to find the roots of a polynomial, solve problems involving the area of a rectangle, and solve problems involving the Pythagorean theorem.
Question 6: What are some common mistakes to avoid when solving quadratic equations by factoring?
Answer: Some common mistakes to avoid when solving quadratic equations by factoring include:
- Not factoring the equation correctly
- Not setting each factor equal to zero
- Not solving each equation for x
- Making errors in the calculations
By avoiding these common mistakes, you can improve your accuracy when solving quadratic equations by factoring.
Summary: Solving quadratic equations by factoring is a valuable skill that can be used to solve a variety of problems. By understanding the steps involved in factoring and solving quadratic equations, you can improve your ability to solve these equations correctly.
Next: Practice Problems
Tips for Solving Quadratic Equations by Factoring
Solving quadratic equations by factoring is a valuable skill that can be used to solve a variety of problems. Here are a few tips to help you master this skill:
Tip 1: Factor the quadratic equation correctly.
The first step in solving a quadratic equation by factoring is to factor the equation. This involves finding two numbers that multiply to give the constant term and add to give the coefficient of the x-term. There are a few different methods for factoring quadratic equations, so it is important to find a method that works for you.
Tip 2: Set each factor equal to zero.
Once you have factored the quadratic equation, you can set each factor equal to zero. This will give you two equations, which you can solve for x.
Tip 3: Solve each equation for x.
Solving each equation for x will give you the two solutions to the original quadratic equation. These solutions can be either real numbers or complex numbers.
Tip 4: Check your answers.
Once you have found the solutions to the quadratic equation, it is important to check your answers. You can do this by plugging your solutions back into the original equation and verifying that they make the equation true.
Tip 5: Practice, practice, practice!
The best way to improve your skills at solving quadratic equations by factoring is to practice. There are many different online and offline resources that can provide you with practice problems.
Summary: Solving quadratic equations by factoring is a valuable skill that can be used to solve a variety of problems. By following these tips, you can improve your accuracy and efficiency when solving quadratic equations.
Next: Practice Problems
Conclusion
Solving quadratic equations by factoring is a valuable skill that can be used to solve a variety of problems. This worksheet has provided you with the tools and practice you need to master this skill.
Remember to practice regularly and don’t be afraid to seek help if you need it. With practice, you will become proficient at solving quadratic equations by factoring and be able to use this skill to solve a variety of problems.
Youtube Video: